ERC Consolidator Grant
About Project
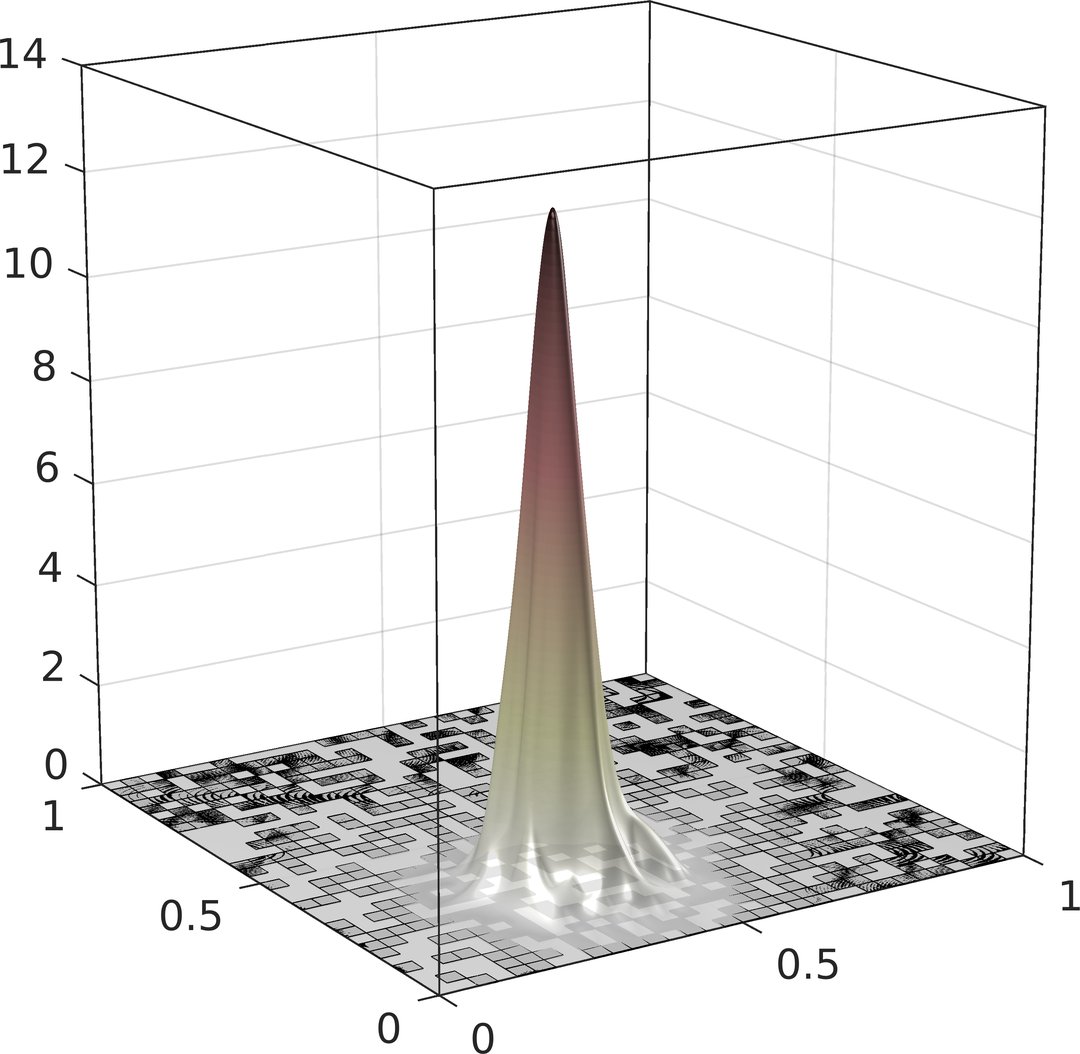
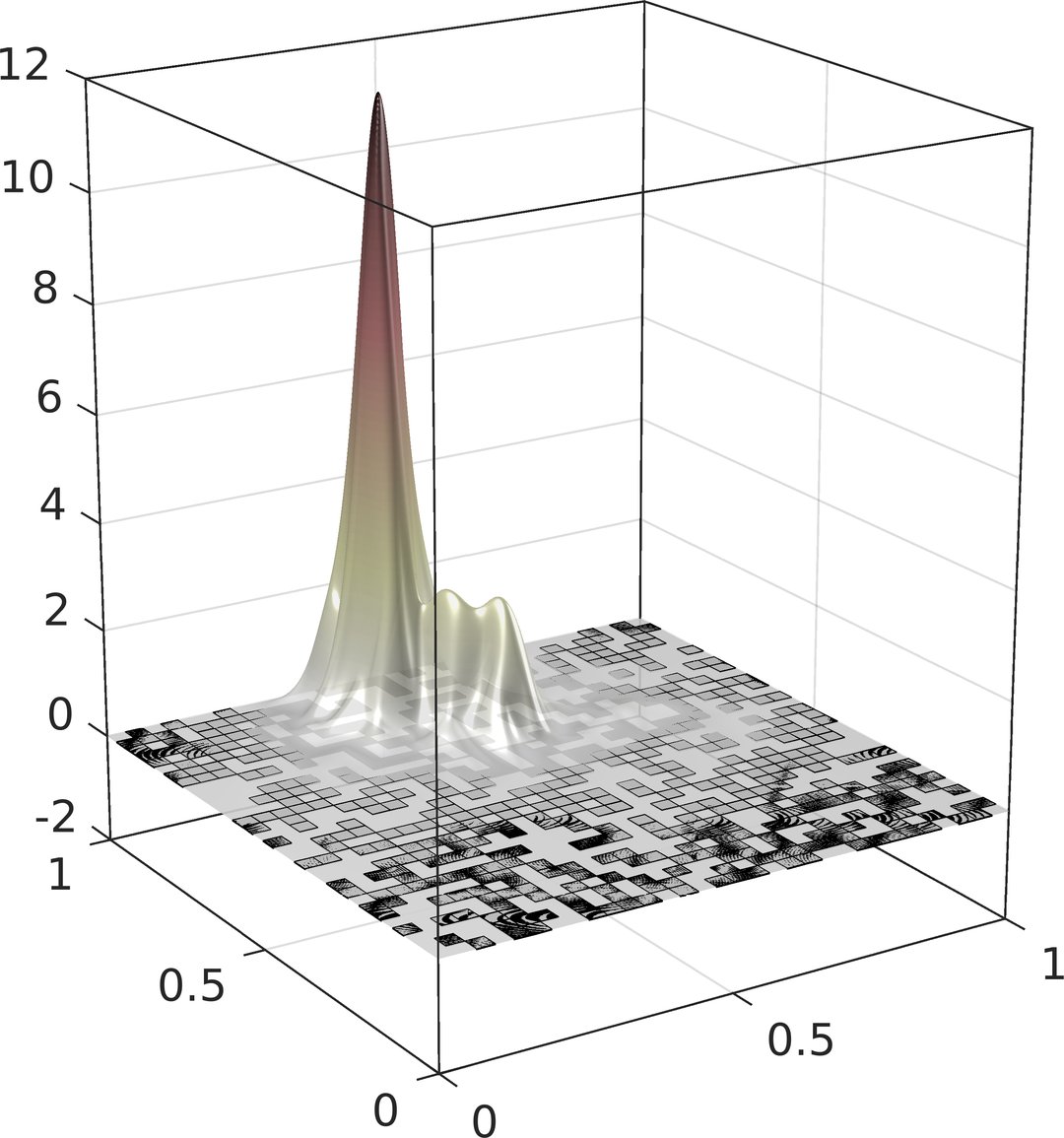
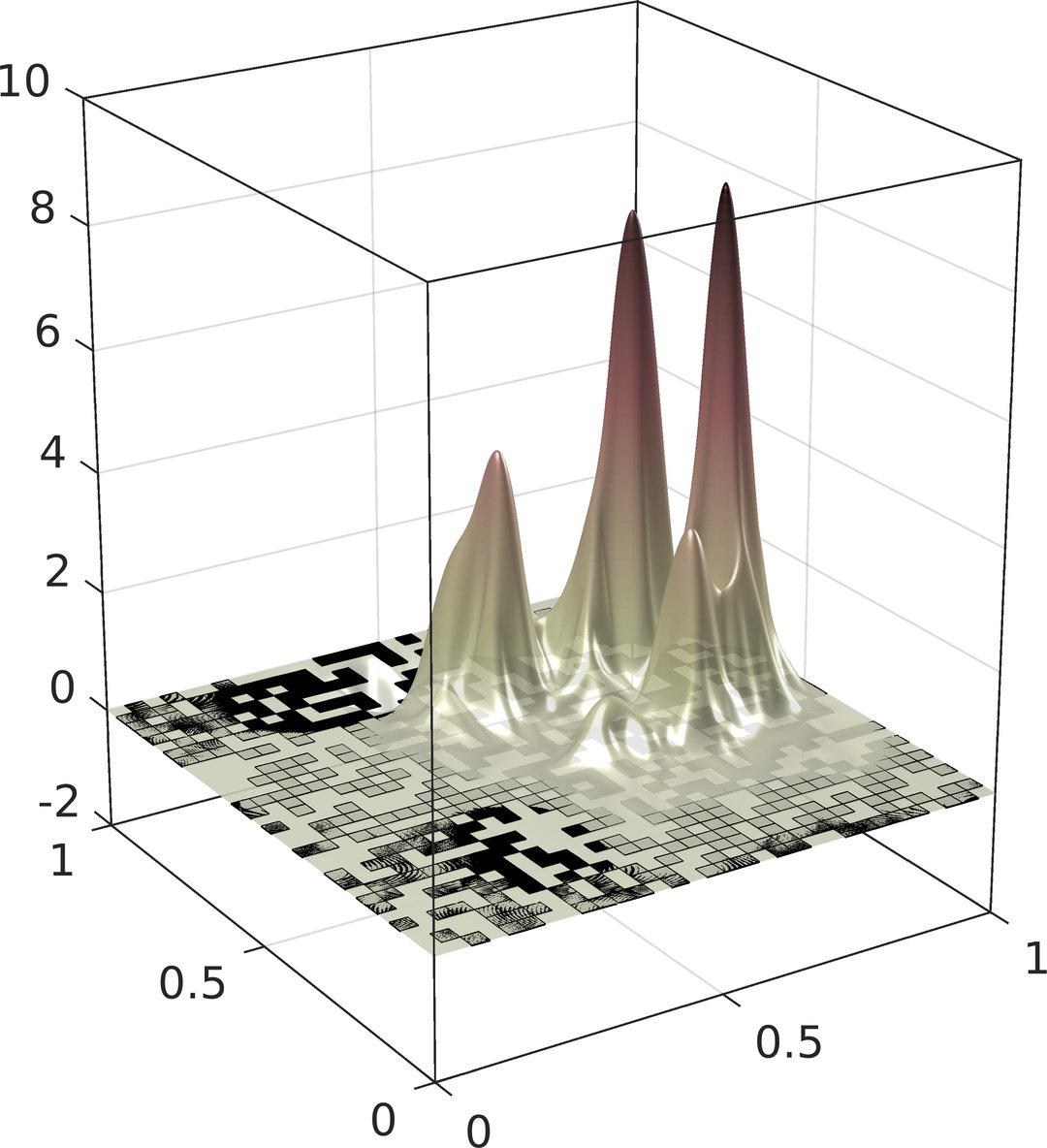
Computational Random Multiscale Problems
PI: Prof. Dr. Daniel Peterseim
Geometrically or statistically heterogeneous microstructures and high physical contrast are the key to astonishing physical phenomena such as invisibility cloaking with metamaterials or the localization of quantum waves in disordered media. Due to the complex experimental observation of such processes, numerical simulation has very high potential for their understanding and control. However, the underlying mathematical models of random partial differential equations are characterized by a complex interplay of effects on many non-separable or even a continuum of characteristic scales. The attempt to resolve them in a direct numerical simulation easily exceeds today's computing resources by multiple orders of magnitude. The simulation of physical phenomena from multiscale models, hence, requires a new generation of computational multiscale methods that accounts for randomness and disorder in a hierarchical and adaptive fashion.
This project concerns the design and numerical analysis of such methods. The main goals are connected to fundamental mathematical and algorithmic challenges at the intersection of multiscale modeling and simulation, uncertainty quantification and computational physics:
(A) Numerical stochastic homogenization beyond stationarity and ergodicity,
(B) Uncertainty quantification in truly high-dimensional parameter space,
(C) Computational multiscale scattering in random heterogeneous media,
(D) Numerical prediction of Anderson localization and quantum phase transitions.
These objectives base upon recent breakthroughs of deterministic numerical homogenization beyond periodicity and scale separation and its deep links to seemingly unrelated theories ranging all the way from domain decomposition to information games and their Bayesian interpretation. It is this surprising nexus of classical and probabilistic numerics that clears the way to the envisioned new computational paradigm for multiscale problems at randomness and disorder.
Community Research and Development Information Service (CORDIS) of the European Commission
Members
Events
Upcoming:
| Follow-Up Workshop to Multiscale Problems, Hausdorff Research Institute for Mathematics, 19.-23. August 2024 |
Previous:
| Workshop on Numerical Analysis of Nonlinear Schrödinger Equations, University of Augsburg, 22. June 2023 |
| Jena-Augsburg-Meeting (JAM) on Numerical Analysis, University of Augsburg, 7.-10. June 2023 |
| Summer School - Uncertainty, Adaptivity, and Machine Learning, University of Augsburg 12.-14. September 2022 |
| Workshop on scattering by random heterogeneous media, University of Augsburg, 13.-15. September 2021 |
Talks
Upcoming:
| European Conference on Numerical Mathematics and Advanced Applications (ENUMATH), Lisbon, 4.-8. September 2023. |
|
Given talks:
|
Publications
Submitted articles:
|
[27]
|
R. Altmann, D. Peterseim, T. Stykel. Riemannian Newton methods for energy minimization problems of Kohn-Sham type, ArXiv e-prints, 2023. [ arXiv ] |
|
|
[26]
|
F. Bonizzoni, K. Hu, G. Kanschat, D. Sap. Discrete tensor product BGG sequences: splines and finite elements, ArXiv e-prints, 2023. [ arXiv ] |
|
|
[25]
|
P. Freese, D. Gallistl, D. Peterseim, T. Sprekeler. Computational multiscale methods for nondivergence-form elliptic partial differential equations, ArXiv e-prints, 2022. [ arXiv ] |
|
|
[24]
|
G. Balduzzi, F. Bonizzoni, L. Tamellini. Uncertainty quantification in timber-like beams using sparse grids:
theory and examples with off-the-shelf software utilization, ArXiv e-prints, 2022. [ arXiv ] |
|
|
[23]
|
F. Bonizzoni, M. Hauck, and D. Peterseim. A reduced basis super-localized orthogonal decomposition for reaction-convection-diffusion problems, ArXiv e-prints, 2022. [ arXiv ] |
|
|
[22]
|
P. Freese, M. Hauck, T. Keil and D. Peterseim. A Super-Localized Generalized Finite Element Method, Arxiv e-prints, 2022. [ arXiv ] |
|
|
[21]
|
Z. Dong, M. Hauck and R. Maier. An improved high-order method for elliptic multiscale problems, Arxiv e-prints, 2022. [ arXiv ] |
|
|
[20]
|
M. Hauck and A.Målqvist. Super-localization of spatial network models, ArXiv e-prints, 2022. [ arXiv ] |
|
|
[19]
|
M. Alghamdi, D. Boffi and F. Bonizzoni. A greedy MOR method for the tracking of eigensolutions to parametric elliptic PDEs. ArXiv e-prints, 2022. [ arXiv ] |
|
|
[18]
|
F. Bonizzoni, P. Freese and D. Peterseim. Super-localized orthogonal decomposition for convection-dominated diffusion problems, ArXiv e-prints, 2022. [ arXiv ] |
|
|
[17]
|
P. Freese, M. Hauck and D. Peterseim. Super-localized Orthogonal Decomposition for high-frequency Helmholtz problems, ArXiv e-prints, 2021. [ arXiv ] |
Refereed articles:
|
[16]
|
F. Bonizzoni, G. Kanschat. A Tensor-Product Finite Element Cochain Complex with Arbitrary Continuity. eccomas2022, 2022. [ DOI ] |
|
|
[15]
|
F. Bonizzoni, D. Pradovera and M. Ruggeri. Rational-based model order reduction of Helmholtz frequency response problems with adaptive finite element snapshots. Math. eng., 5(4): 1-38, 2023. |
|
|
[14]
|
P. Freese. The Heterogeneous Multiscale Method for dispersive Maxwell systems. SIAM Multiscale Modeling & Simulation, 20(2): 769-797, 2022. [ DOI ] |
|
|
[13]
|
R. Altmann, D. Peterseim and T. Stykel. Energy-adaptive Riemannian optimization on the Stiefel manifold. ESAIM: M2AN, 56(5): 1629-1653, 2022. |
|
|
[12]
|
F. Kröpfl, R. Maier and D. Peterseim. Operator compression with deep neural networks. Adv Cont Discr Mod 2022, 29 (2022). |
|
|
[11]
|
M. Hauck and D. Peterseim. Super-localization of elliptic multiscale problems. Mathematics of Computation, 92: 981-1003, 2023. |
|
|
[10]
|
M. Hauck and D. Peterseim. Multi-resolution Localized Orthogonal Decomposition for Helmholtz problems. SIAM Multiscale Modeling & Simulation, 20(2): 657-684, 2022. [ DOI ] |
|
|
[9]
|
R. Altmann, P. Henning and D. Peterseim. Localization and delocalization of ground states of Bose-Einstein condensates under disorder. SIAM J. Appl. Math., 82, 330-358, 2022. [ arXiv | DOI ] |
|
|
[8]
|
R. Altmann, P. Henning and D. Peterseim. The J-method for the Gross-Pitaevskii eigenvalue problem. Numerische Mathematik, 148(3): 575-610, 2021. [ arXiv | DOI ] |
|
|
[7]
|
R. Altmann, P. Henning and D. Peterseim. Numerical homogenization beyond scale separation. Acta Numerica, pp. 1-86, 2021. [ DOI ] |
|
|
[6]
|
J. Fischer, D. Gallistl and D. Peterseim. A priori error analysis of a numerical stochastic homogenization method. SIAM J. Numer. Anal., 59(2): 660-674, 2021. [ arXiv | DOI ] |
Monograph:
|
[5]
|
A. Målqvist and D. Peterseim. Numerical homogenization by localized orthogonal decomposition. SIAM Spotlights 5, ISBN: 978-1-611976-44-1, 2020. [ SIAM ] |
Preliminary work
|
[4]
|
M. Feischl and D. Peterseim. Sparse compression of expected solution operators. SIAM J. Numer. Anal., 58(6):3144-3164, 2020. |
|
[3]
|
P. Henning and D. Peterseim. Sobolev gradient flow for the Gross-Pitaevskii eigenvalue problem: global convergence and computational efficiency. SIAM J. Numer. Anal., 58(3):1744–1772, 2020. |
|
[2]
|
R. Altmann, P. Henning, and D. Peterseim. Quantitative Anderson localization of Schrödinger eigenstates under disorder potentials. M3AS Math. Models Methods Appl. Sci., 30(5):917-955, 2020. |
|
[1]
|
D. Peterseim and B. Verfürth. Computational high frequency scattering from high contrast heterogeneous media. Math. Comp., 89:2649-2674, 2020. [ arXiv | DOI ] |